Table of Links
-
Proposed Approach
C. Formulation of MLR from the Perspective of Distances to Hyperplanes
H. Computation of Canonical Representation
3.3 MLR IN STRUCTURE SPACES
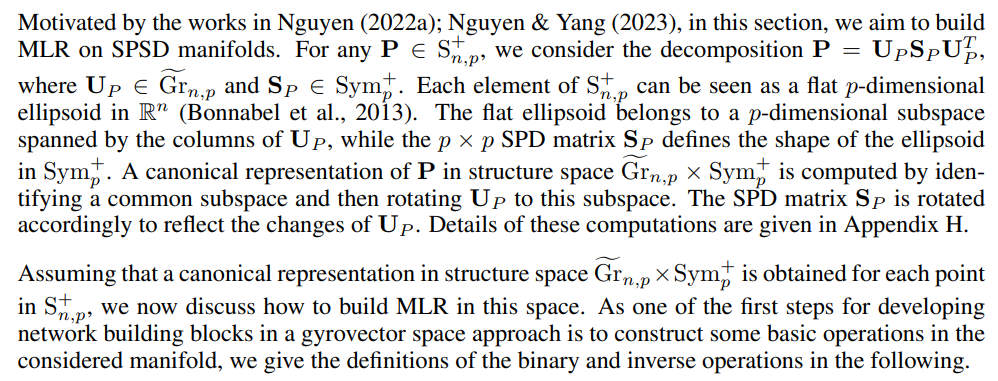
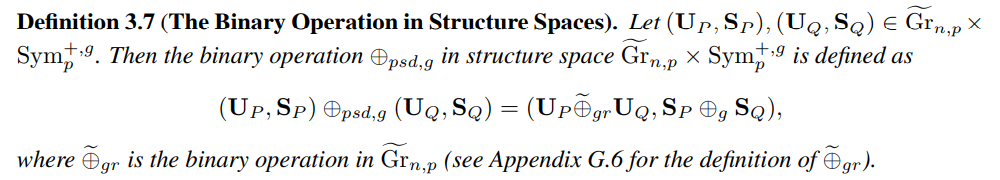
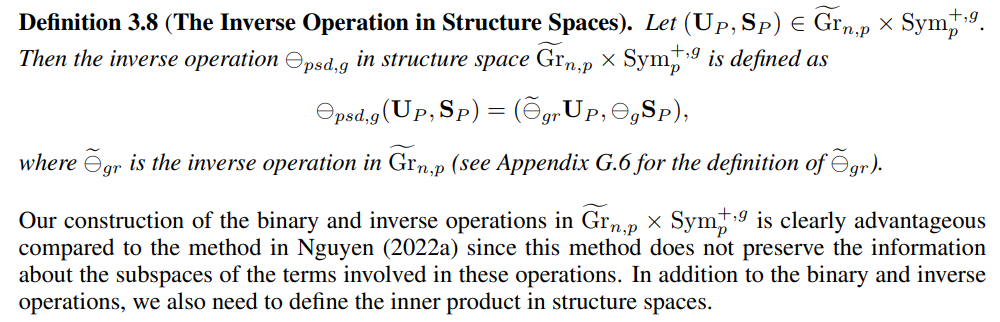
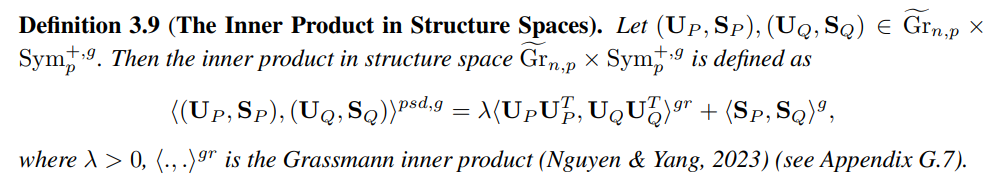
The key idea to generalize MLR to a Riemannian manifold is to change the margin to reflect the geometry of the considered manifold (a formulation of MLR from the perspective of distances to hyperplanes is given in Appendix C). This requires the notions of hyperplanes and margin in the considered manifold that are referred to as hypergyroplanes and pseudo-gyrodistances (Nguyen & Yang, 2023), respectively. In our case, the definition of hypergyroplanes in structure spaces, suggested by Proposition 3.2, can be given below.
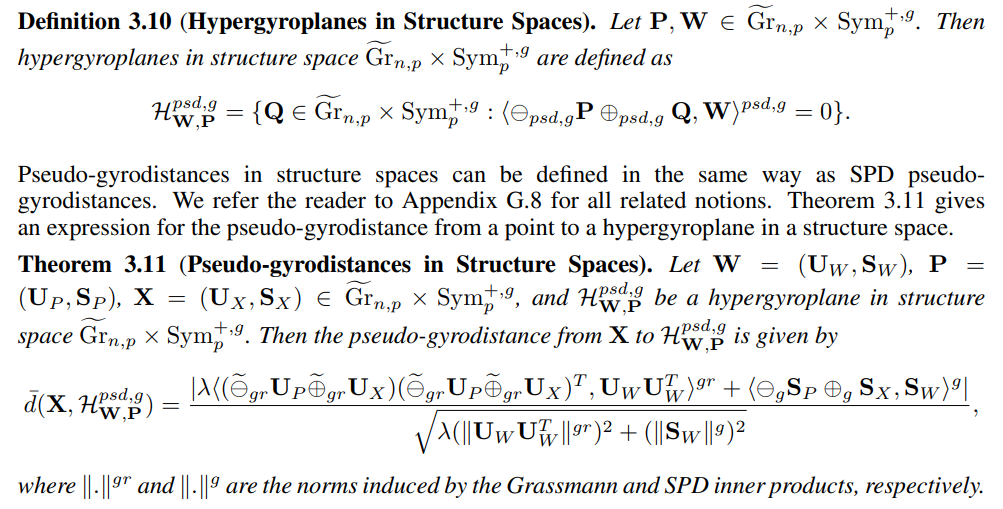
Proof See Appendix M.
The algorithm for computing the pseudo-gyrodistances is given in Appendix B.
Authors:
(1) Xuan Son Nguyen, ETIS, UMR 8051, CY Cergy Paris University, ENSEA, CNRS, France ([email protected]);
(2) Shuo Yang, ETIS, UMR 8051, CY Cergy Paris University, ENSEA, CNRS, France ([email protected]);
(3) Aymeric Histace, ETIS, UMR 8051, CY Cergy Paris University, ENSEA, CNRS, France ([email protected]).
This paper is

